This chapter has two methods of cubing.
- Method 1: Cubing by the ratio method
- Method 2: Cubing by general formula
Before proceeding to the methods the student should memorize the cubes of first 10 natural numbers stated below.
13 = 1
23 = 8
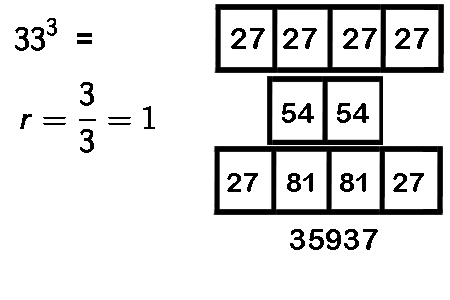
33 = 27
43 = 64
53 = 125
63 = 216
73 = 343
83 = 512
93 = 729
103 = 1000
Method 1: Cubing by the ratio method
Example 1 : 123
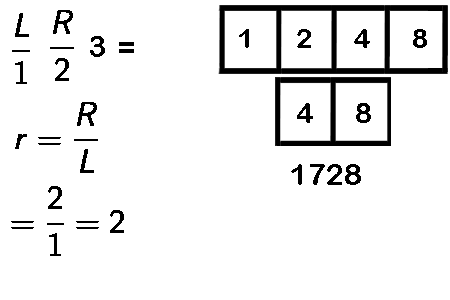
Step 1: Make 4 boxes. Write the cube of left digit in the left box 13 = 1
Step 2: Calculate the ratio of right to left digit ratio r = R/L = 2/1 = 2
Step 3: Multiply the number in left box by this ratio r and keep on subsequently multiplying the numbers obtained in the boxes, by same ratio till you reach the last (IVth) box
Step 4: Double the numbers in the second and third box and write below them
Step 5: Add up the numbers box wise from right to left and carry over wherever required to obtain the final answer as 1728
Example 2 :
Example 3 :

Example 4 :
Example 5 :

Exercises:
153 = 15625
273 = 19683
223 = 10648
353 = 42875
513 = 132651
653 = 166375
133 = 2197
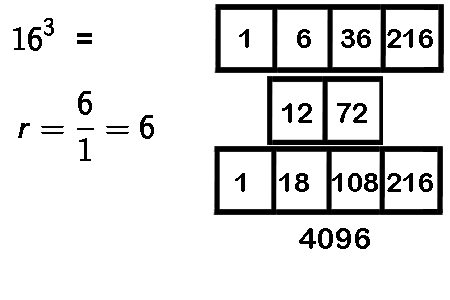
163 = 4096
193 = 6859
973 = 912673
993 = 970299
Method 2: Cubing by using general formula
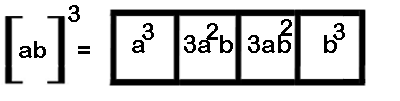
Example 1 :
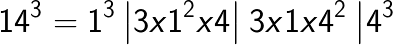
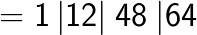
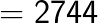 (Note the carry over from the right boxes to the left boxes)
(Note the carry over from the right boxes to the left boxes)
Example 2 :
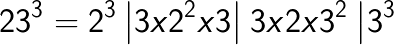
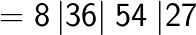
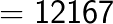
Example 3 :
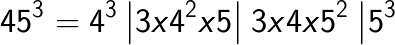
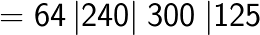
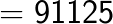
Exercises:
113 = 1331
173 = 4913
183 = 5832
263 = 17576
253 = 15625
373 = 50653
423 = 74088
523 = 140608
633 = 250047
753 = 421875
813 = 531441
983 = 941192
Corollary:
We can extend the general formula to calculate the cube of three digit numbers as well.

Example 1: 1053 (Here we need to substitute a = 10 and b = 5 in the above formula).
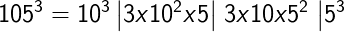

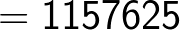
Example 2: 1033
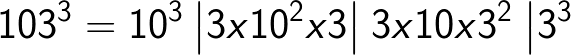
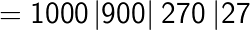
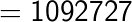
Example 3: 1023
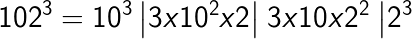
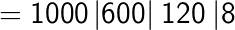
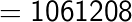
Course:


