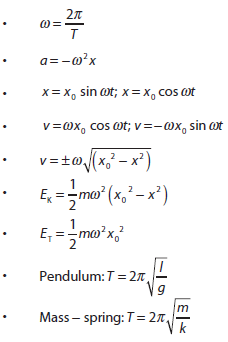|
Nature of science:
Insights: The equation for simple harmonic motion (SHM) can be solved analytically and numerically. Physicists use such solutions to help them to visualize the behaviour of the oscillator. The use of the equations is very powerful as any oscillation can be described in terms of a combination of harmonic oscillators. Numerical modelling of oscillators is important in the design of electrical circuits. (1.11)
|
Understandings:
- The defining equation of SHM
- Energy changes
Applications and skills:
- Solving problems involving acceleration, velocity and displacement during simple harmonic motion, both graphically and algebraically
- Describing the interchange of kinetic and potential energy during simple harmonic motion
- Solving problems involving energy transfer during simple harmonic motion, both graphically and algebraically
Guidance
- Contexts for this sub-topic include the simple pendulum and a mass-spring system
|
Utilization:
- Fourier analysis allows us to describe all periodic oscillations in terms of simple harmonic oscillators. The mathematics of simple harmonic motion is crucial to any areas of science and technology where oscillations occur
- The interchange of energies in oscillation is important in electrical phenomena
- Quadratic functions (see Mathematics HL sub-topic 2.6; Mathematics SL sub- topic 2.4; Mathematical studies SL sub-topic 6.3)
- Trigonometric functions (see Mathematics SL sub-topic 3.4)
|
|
Data booklet reference:
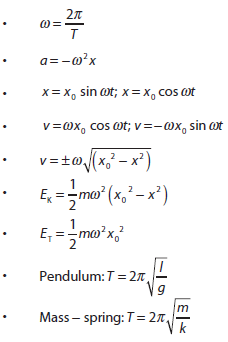
|
Aims:
- Aim 4: students can use this topic to develop their ability to synthesize complex and diverse scientific information
- Aim 6: experiments could include (but are not limited to): investigation of simple or torsional pendulums; measuring the vibrations of a tuning fork; further extensions of the experiments conducted in sub-topic 4.1. By using the force law, a student can, with iteration, determine the behaviour of an object under simple harmonic motion. The iterative approach (numerical solution), with given initial conditions, applies basic uniform acceleration equations in successive small time increments. At each increment, final values become the following initial conditions.
- Aim 7: the observation of simple harmonic motion and the variables affected can be easily followed in computer simulations
|